We take our number system for granted. All around the world, if someone writes 647, it is understood to mean six hundred forty seven. We have ten digits and a placeholder system, and we can not only write any whole number, we can write negative numbers and numbers with decimal and many more variations. This system is known as the Hindu-Arabic numeral system, and it has become the worldwide standard because of its ease of use and versatility. But if we go about 500 years back in Europe, only a few educated people would know about the Hindu-Arabic system and most people would be using Roman numerals.
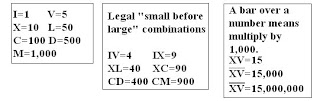
Roman numerals had a total of seven symbols, which were letters used in nearly every European alphabet, I, V, X, L, C, D and M, and another non-letter symbol, a bar placed over a number. The bar over letters meant to take those letters, figure out what numbers they stood form then multiply that by 1,000.
The symbols stood for numerical values, and when they were written the symbols were put in order left to right with the largest symbols first down to the smallest symbols. There were a few legal combinations where a small number symbol could be put before a big number symbol, and the rule were as follows.
Rule 1: The small number symbol must represent a power of 10, I for 1, X for 10 or C for 100.
Rule 2: The large number symbol that followed the small must be either five times larger or ten times larger. The six possible combinations are list in the picture above.
If we wanted to represent the number 642,318 in Roman numerals, we would have the symbols for 642 under a bar followed by the symbols for 318. It would look like this.
______
DCXLIICCCXVIII
Below, the symbols are put in red and black to show which groups of symbols represent which digit in the number 642,318.
______
DCXLIICCCXVIII
The rules are that if we want to represent a number in the hundreds place, we look for the first instance of a D or C. For the tens place, we look for the first instance of a L or X, and the ones place starts when we see the first instance of a I or V.
While reading and writing Roman numerals can be taught fairly easily, addition and subtraction rules are a little cumbersome, while multiplication and division are truly difficult. Because of this, Roman numerals have disappeared as a way to represent numbers except in ornamental ways, like Super Bowls and fancy clock numbers.
Fractions vs. Decimals
Fractions and decimals are two different ways to represent the same types of numbers. Both methods have advantages and disadvantages, so unlike Roman numerals, neither method is likely to go away soon.
Mathematicians like fractions because they are precise. When I write 5/13, this means the exact number that when I multiply it by 13, I get 5. If you put 5/13 in a calculator, the readout will be 0.384615385, and that's just the first nine digits after the decimal place. The true representation goes on forever, but because it is a rational number it has a pattern that repeats. The standard way to write a repeating decimal is to put a bar over the repeating part so in this instance we have
_______
.384615 = 5/13
One of the drawbacks of fractions is that if they have different denominators, it can be hard to tell which is larger. Foe example, is 5/11 larger than 4/9? It's hard to tell looking at them, but if we find a common denominator, like 11x9 = 99, it becomes clear.
(5x9)/(11x9)= 45/99
(4x11)/(9x11)=44/99
In this case 5/11 is greater than 4/9. If we went to our calculators and found the decimal representations, it would be very clear immediately.
5/11 = .45454545...
___
.45 = 5/11
4/9=.4444...
__
.4= 4/9
Since the first time the number disagree, the 5 in the hundredths place is bigger than the 4 in the hundredths place, that means 5/11 is the larger of the two numbers.
It's important to realize that any time we round a decimal number off and we lose information, we do not get a true exact value of a fraction. Some fractions terminate, but only if the denominator is only divisible by 2 or 5.

No comments:
Post a Comment